Cube Counting for the DAT
/Learn key cube counting concepts and strategy, plus practice questions and answers
Table of Contents
Part 1: Introduction to cube counting
Part 2: Rules and strategies
a) Rules
b) Strategies
Part 3: Questions and answers
----
Part 1: Introduction to cube counting
The cube counting section of the PAT can seem daunting. There are numerous cubes with many sides that aren’t visible, and you have to determine how many cubes have only a certain number of sides exposed. It will likely take you a minute or two just to count all of the cubes, and with only 60 minutes to answer 90 PAT questions, time is limited!
If you are worried about this portion of the PAT, rest assured that with a proper understanding of this section, an effective strategy, and practice, you can ace cube counting and fly through the questions. This guide will go over the rules of cube counting and effective strategies. We will walk you through practice problems, and there are questions and answers at the end to enhance your learning.
----
Part 2: Rules and strategies
a) Rules
Cube counting is the fifth section of the PAT. You have 60 minutes to complete 6 PAT sections, so you’ll have about 10 minutes to complete the cube counting section. There will be 15 cube counting questions that follow this format:
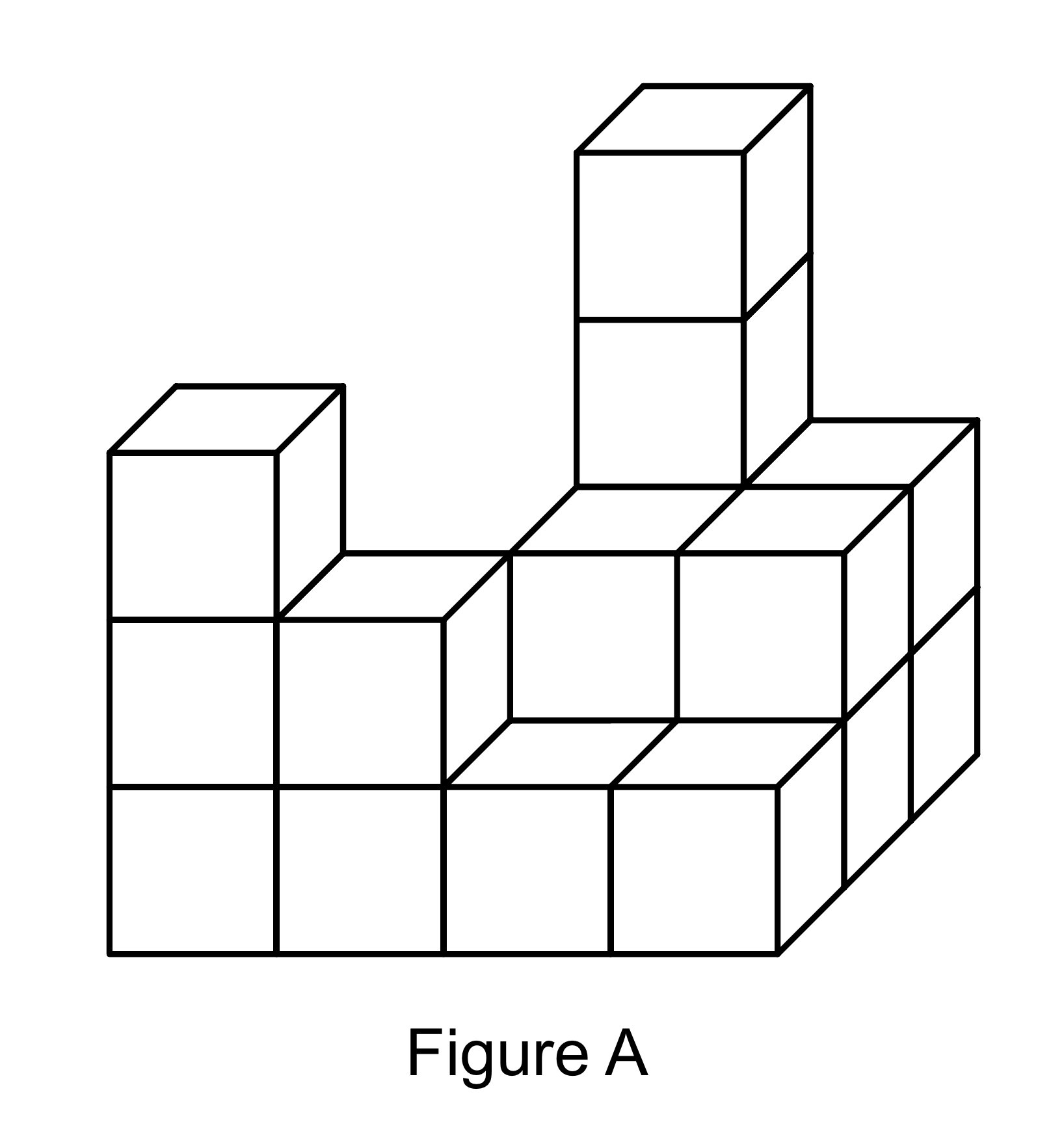
In Figure A, how many cubes have # of their exposed sides painted?
a) 0 cubes
b) 1 cube
c) 2 cubes
d) 3 cubes
e) 4 cubes
After reading through this question, you might be wondering why it’s asking how many sides are painted. The cube counting section requires you to count the number of exposed sides on each cube. Although the diagram is black and white, it is implied that the exposed sides of each cube are painted.
A cube has six sides, but the bottom side will usually be resting on the ground or another cube. All cubes will be connected by at least one side to each other. This means that there will never be more than five sides of a cube exposed.
Cubes are set on top of or next to each other. Sides of cubes that are next to each other are no longer exposed. The same goes for sides of cubes that are stacked. Here is an example of cubes stacked next to and on top of each other. Try your best to count how many exposed sides each of the cubes has, mentally rotating the stack to visualize each side.
Now that you’ve had some time to visualize it yourself, let’s walk through this example together. In the following figures, each side of each cube is numbered. The second figure shows the stack of cubes rotated counterclockwise, and the third figure depicts a 180° rotation.
The left cube has a total of 4 exposed sides. The top right cube has 5 painted sides, and the bottom right cube has 3.
Now that you have a basic understanding of how to count cubes, let’s go over the rules of this section. Understanding the rules will help you make sense of tricky figures.
Cubes may be hidden from view, but there will never be hidden cubes without some indication.
Gain instant access to the most digestible and comprehensive DAT content resources available. Subscribe today to lock in the current investments, which will be increasing in the future for new subscribers.